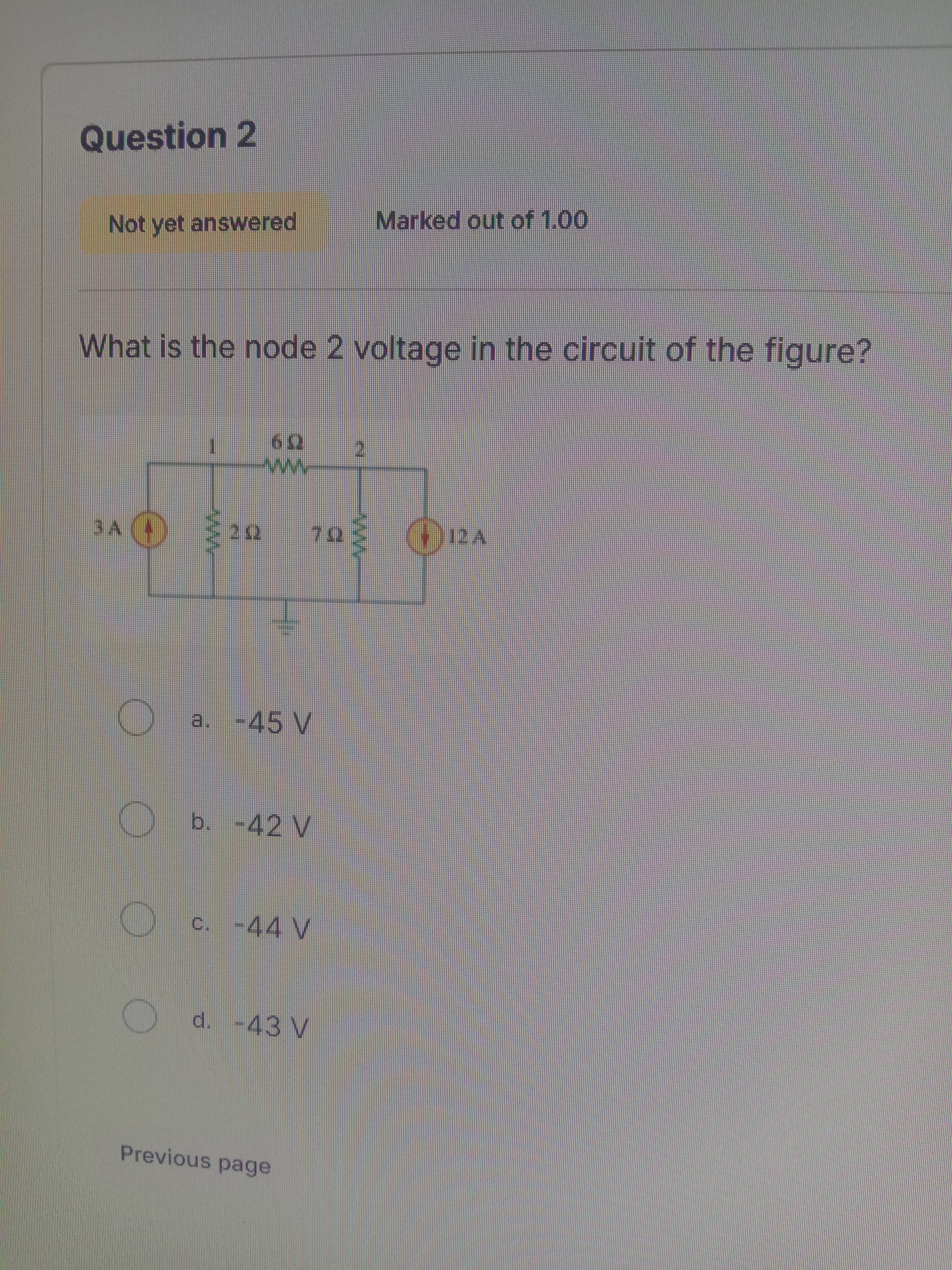
What is the node 2 voltage in the circuit of the figure?

Steps to Solve

1. Identify the given values The circuit has the following values:

• Current $I_1 = 3 , \text{A}$
• Current $I_2 = 12 , \text{A}$
• Resistances are $R_1 = 6 , \Omega$, $R_2 = 2 , \Omega$, and $R_3 = 7 , \Omega$.

2. Apply Kirchhoff's Current Law (KCL) at Node 2 At Node 2, the incoming current is equal to the outgoing currents: $$ I_2 = I_{R_2} + I_{R_3} $$ Thus, we can write: $$ 12 = \frac{V_2 - V_1}{R_2} + \frac{V_2 - 0}{R_3} $$

3. Relate voltages using Ohm's Law From Ohm's Law, we can express the currents through $R_2$ and $R_3$: $$ I_{R_2} = \frac{V_2 - V_1}{2} $$ $$ I_{R_3} = \frac{V_2}{7} $$

Substituting these in gives: $$ 12 = \frac{V_2 - V_1}{2} + \frac{V_2}{7} $$

4. Solve for voltage $V_2$ To solve the equation, find a common denominator (14) and rewrite the equation: $$ 12 = \frac{7(V_2 - V_1) + 2V_2}{14} $$ Expanding yields: $$ 12 * 14 = 7(V_2 - V_1) + 2V_2 $$ This simplifies to: $$ 168 = 7V_2 - 7V_1 + 2V_2 $$ Combining like terms gives: $$ 168 = 9V_2 - 7V_1 $$

5. Substitute $V_1$ and solve for $V_2$ From the first node (Node 1): $$ I_1 = \frac{V_1 - 0}{6} $$ Thus, $$ 3 = \frac{V_1}{6} $$ This means: $$ V_1 = 18 , \text{V} $$

Now substitute $V_1$ back into the equation for $V_2$: $$ 168 = 9V_2 - 7(18) $$ This simplifies to: $$ 168 = 9V_2 - 126 $$ $$ 9V_2 = 294 $$ $$ V_2 = 32 , \text{V} $$

6. Adjust for polarity If the reference node is at a higher potential than Node 2, we adjust: $$ V_2 = -32 , \text{V} $$