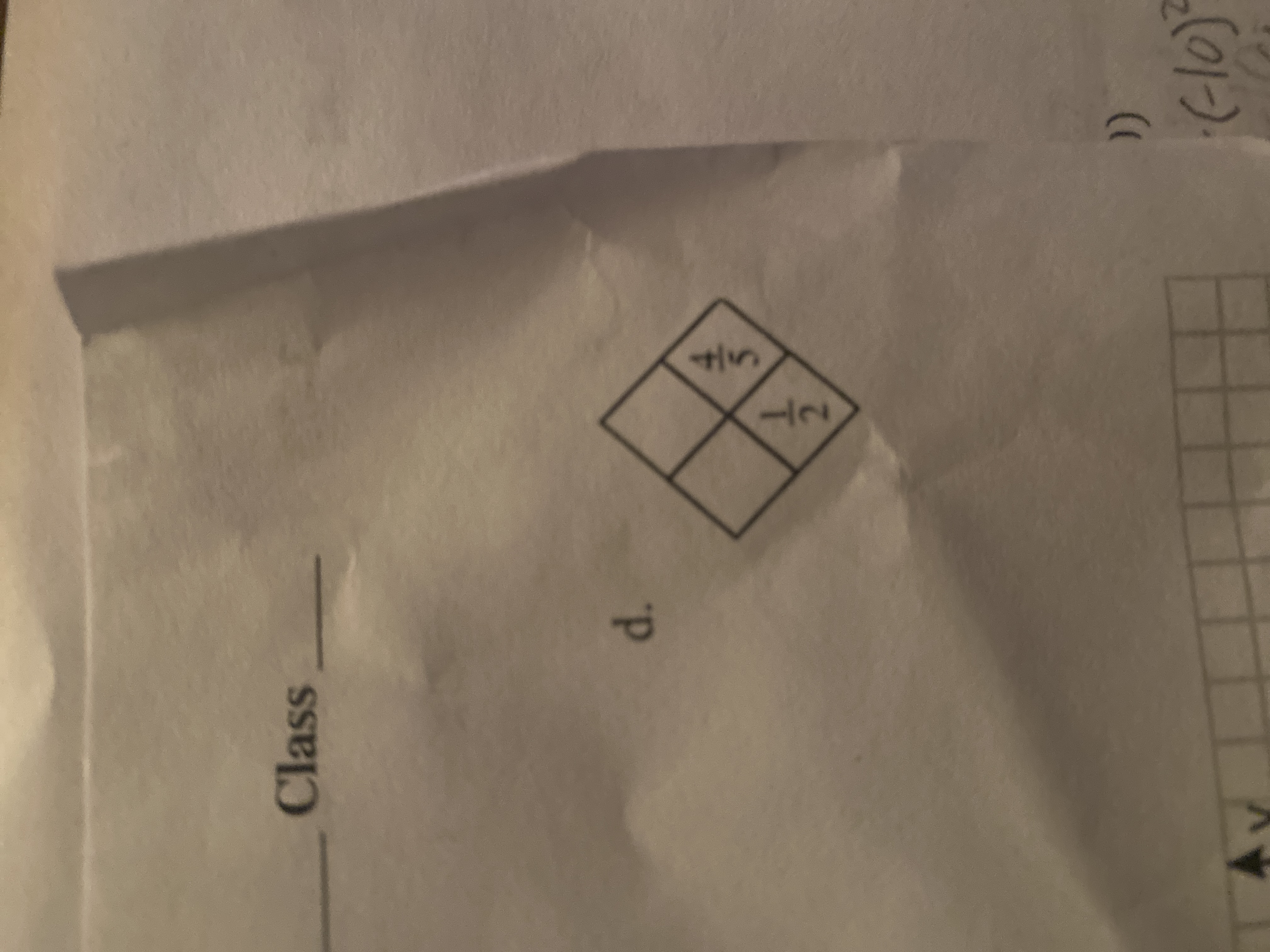
What can you do with the fractions 4/5 and 1/2 shown in the diamond shape?
Understand the Problem
The question appears to be related to interpreting a visual representation of a diamond shape containing the numbers 4/5 and 1/2. The user is likely being asked to perform a specific mathematical operation involving these fractions, possibly adding, subtracting, or comparing them.
Answer
The relationship is \( \frac{4}{5} > \frac{1}{2} \).
Answer for screen readers
The answer is ( \frac{4}{5} > \frac{1}{2} ).
Steps to Solve
-
Identify the fractions The fractions given are ( \frac{4}{5} ) and ( \frac{1}{2} ).
-
Finding a common denominator To compare the fractions, we need a common denominator. The denominators are 5 and 2. The least common multiple (LCM) of 5 and 2 is 10.
-
Convert the fractions Convert both fractions to have the common denominator of 10:
-
For ( \frac{4}{5} ): $$ \frac{4}{5} = \frac{4 \times 2}{5 \times 2} = \frac{8}{10} $$
-
For ( \frac{1}{2} ): $$ \frac{1}{2} = \frac{1 \times 5}{2 \times 5} = \frac{5}{10} $$
-
Compare the fractions Now that we have both fractions as ( \frac{8}{10} ) and ( \frac{5}{10} ), we can compare them. Since ( 8 > 5 ), we have ( \frac{4}{5} > \frac{1}{2} ).
-
Conclude the comparison The final result shows that ( \frac{4}{5} ) is greater than ( \frac{1}{2} ).
The answer is ( \frac{4}{5} > \frac{1}{2} ).
More Information
When comparing fractions, it is useful to express them with a common denominator to easily see which is larger. This method helps clarify relationships between different fractions.
Tips
- Not finding a common denominator: Many people forget to find a common denominator when comparing fractions, which can lead to incorrect conclusions.
- Incorrect simplification: Ensure when converting fractions that the operations performed on the numerator and denominator are consistent.
AI-generated content may contain errors. Please verify critical information