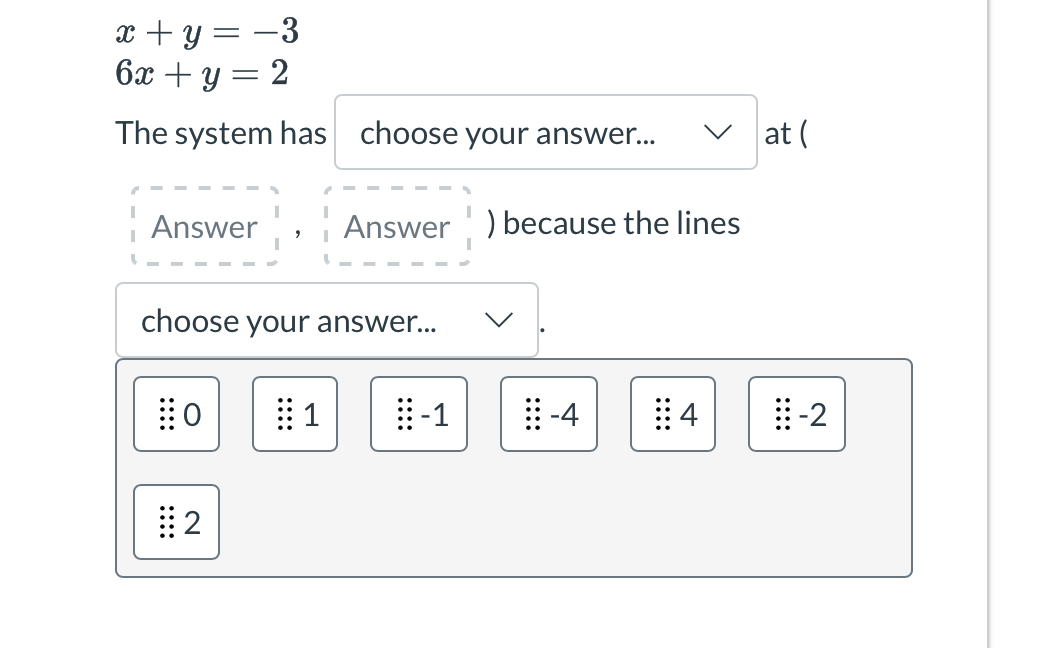
The system has choose your answer at (Answer, Answer) because the lines choose your answer.
Understand the Problem
The question is asking to determine the solution of a given system of linear equations, including where it intersects and its characteristics (e.g. whether the lines are parallel, intersecting, or coincident).
Answer
The system has the solution at \( (1, -4) \) because the lines intersect.
Answer for screen readers
The system has the solution at ( (1, -4) ) because the lines intersect.
Steps to Solve
- Write the equations clearly
The given equations are:
-
( x + y = -3 )
-
( 6x + y = 2 )
-
Solve the first equation for ( y )
Rearranging the first equation gives:
$$ y = -x - 3 $$
- Substitute into the second equation
Substitute ( y ) from the first equation into the second equation:
$$ 6x + (-x - 3) = 2 $$
- Simplify the equation
Now simplify the equation:
$$ 6x - x - 3 = 2 $$
This becomes:
$$ 5x - 3 = 2 $$
- Solve for ( x )
Add 3 to both sides:
$$ 5x = 5 $$
Now divide by 5:
$$ x = 1 $$
- Substitute ( x ) back to find ( y )
Now substitute ( x = 1 ) back into the equation ( y = -x - 3 ):
$$ y = -1 - 3 = -4 $$
- State the intersection point
The solution, or the point where the two lines intersect, is:
$$ (1, -4) $$
- Determine the relationship between the lines
The lines are not parallel since they intersect at a single point. They are therefore considered intersecting lines.
The system has the solution at ( (1, -4) ) because the lines intersect.
More Information
The solution point ( (1, -4) ) represents the coordinates where both equations are satisfied simultaneously. In general, a system of equations can have one solution (intersecting), infinitely many solutions (coincident lines), or no solution (parallel lines).
Tips
- Mistaking the relationship of the lines. Ensure to check if they are parallel or if they intersect.
- Incorrectly substituting values when solving for ( y ). Always double-check each substitution step.
AI-generated content may contain errors. Please verify critical information