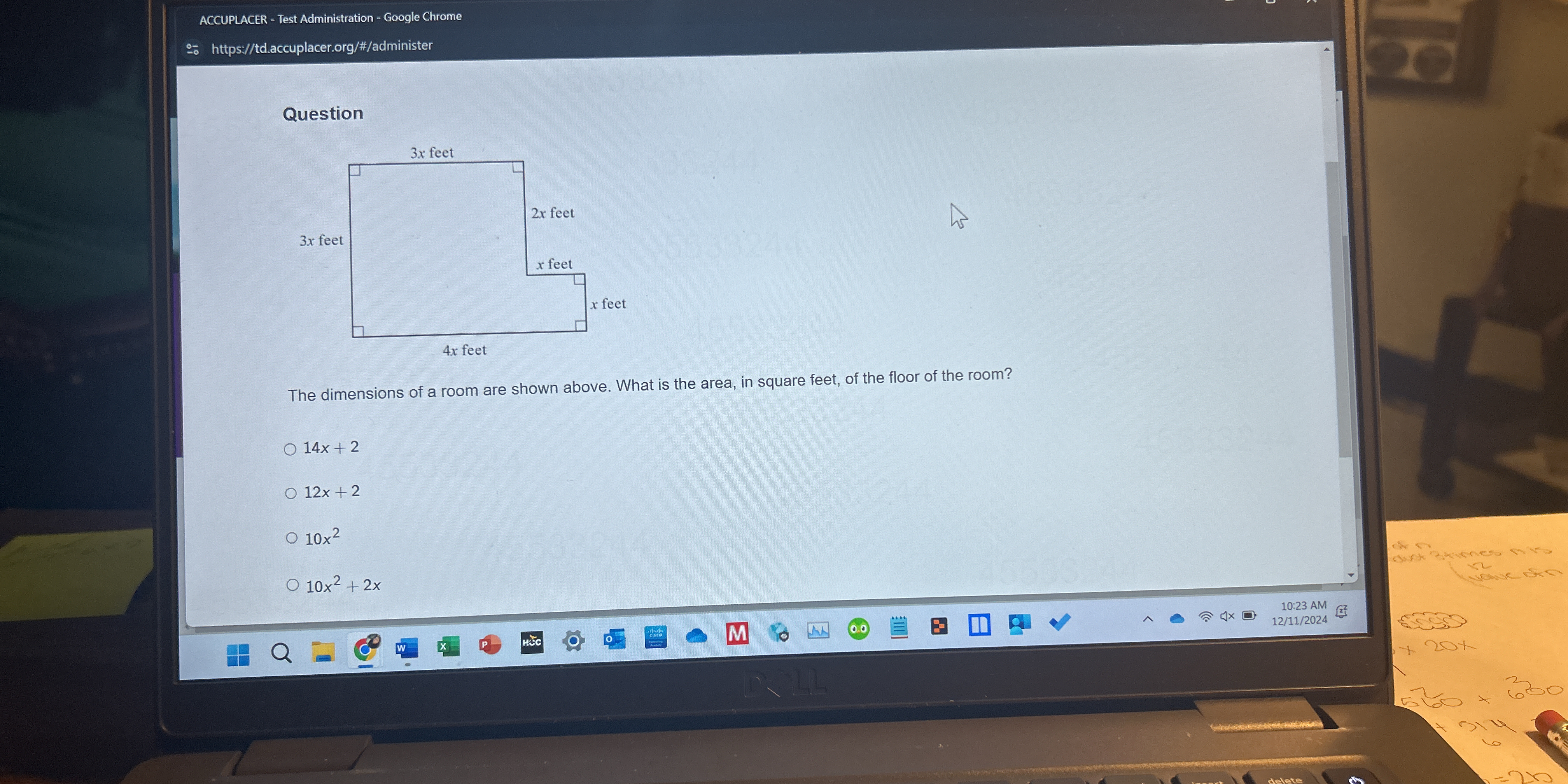
The dimensions of a room are shown above. What is the area, in square feet, of the floor of the room?
Understand the Problem
The question is asking for the area of a room based on given dimensions that can be expressed in terms of variables. To solve it, we will need to calculate the area by multiplying the length and width, and express the final answer appropriately.
Answer
The area of the floor of the room is $12x + 8$ square feet.
Answer for screen readers
The area of the floor of the room is $12x + 8$ square feet.
Steps to Solve
-
Identify the dimensions of the room The room has the following dimensions:
- Width = $3x + 2$ feet (top side)
- Length = $4$ feet (left side)
- Height = $x$ feet (bottom left)
-
Calculate the area of the room The area of a rectangle is given by the formula: $$ A = \text{Length} \times \text{Width} $$
In this case, we will use the dimensions:
- Length = $4$ feet
- Width = $3x + 2$ feet
Substitute these values into the formula: $$ A = 4 \times (3x + 2) $$
-
Distribute to find the area Distribute the $4$: $$ A = 4 \times 3x + 4 \times 2 $$ Simplifying gives: $$ A = 12x + 8 $$
The area of the floor of the room is $12x + 8$ square feet.
More Information
This means that the area can be expressed in a linear form depending on the variable $x$. Depending on the value of $x$, the area will vary, but it always retains the same relationship.
Tips
- Forgetting to distribute the multiplier across both terms when calculating the area.
- Mislabeling the dimensions of the room leading to an incorrect area calculation.
AI-generated content may contain errors. Please verify critical information