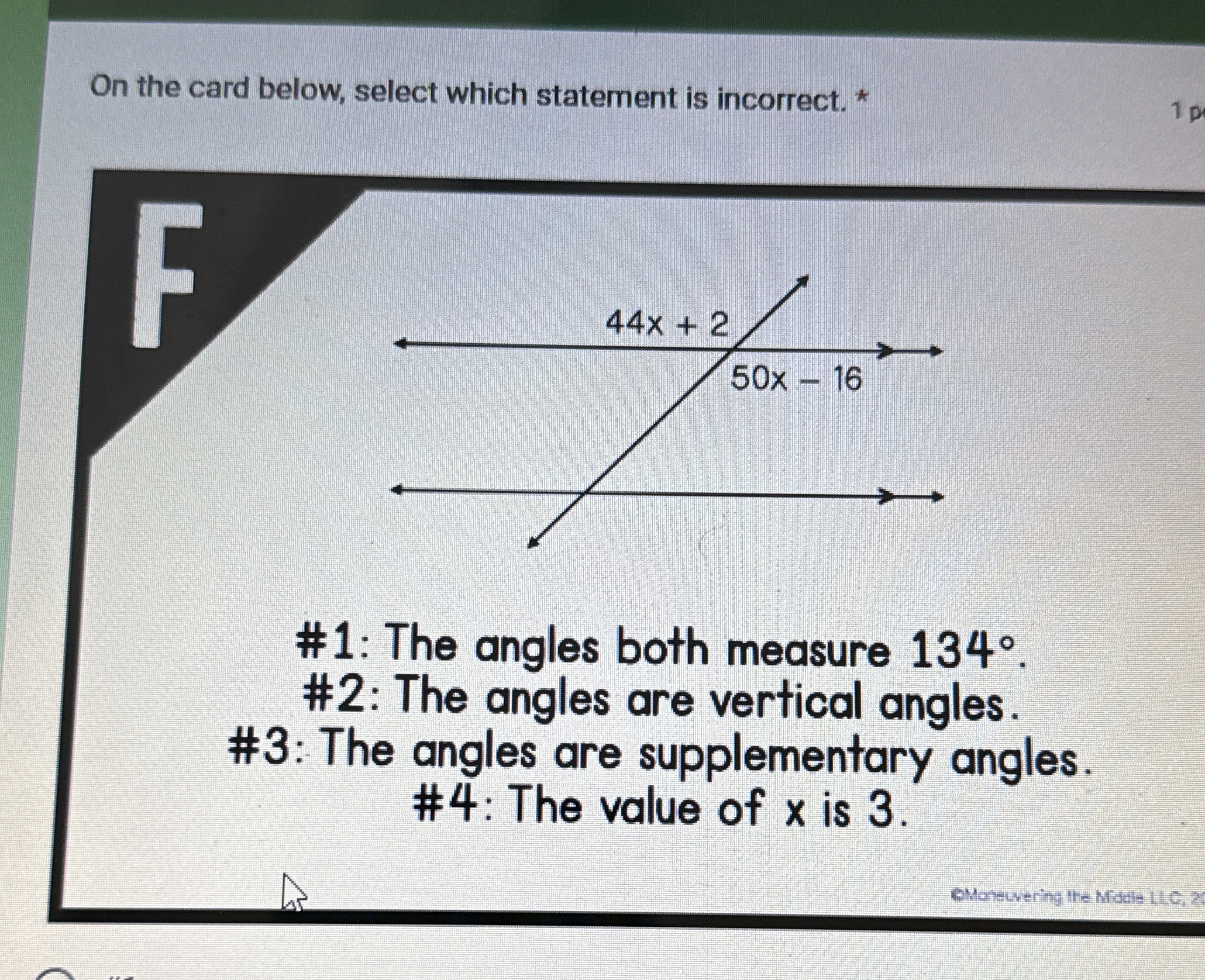
On the card below, select which statement is incorrect: #1: The angles both measure 134°. #2: The angles are vertical angles. #3: The angles are supplementary angles. #4: The value... On the card below, select which statement is incorrect: #1: The angles both measure 134°. #2: The angles are vertical angles. #3: The angles are supplementary angles. #4: The value of x is 3. Read more

Steps to Solve

1. Set up the equation for the angles The angles formed by the intersecting lines are given in terms of $x$: one angle is represented as $44x + 2$ and the other as $50x - 16$.

2. Determine the relationship of the angles Since the lines intersect, we know that the angles can either be supplementary (add up to $180^\circ$) if they are adjacent, or they can be equal if they are vertical angles.

3. Set up the equation for supplementary angles Assuming these angles are supplementary: $$ (44x + 2) + (50x - 16) = 180 $$

4. Combine like terms Combine the $x$ terms and the constants: $$ 94x - 14 = 180 $$

5. Solve for $x$ Add 14 to both sides: $$ 94x = 194 $$

Now divide by 94: $$ x = \frac{194}{94} = 2.065 $$

6. Evaluate the value of x Since $x \approx 2.065$, which is not equal to 3 (as stated in statement #4), that statement is incorrect.

7. Check the angles for correctness Substituting $x = 2.065$ back into the angle expressions:

• For angle 1: $$ 44(2.065) + 2 \approx 92.86^\circ $$
• For angle 2: $$ 50(2.065) - 16 \approx 92.86^\circ $$ Both angles are approximately $92.86^\circ$, confirming that they are vertical angles, consistent with statement #2.