If α > 1 > |w1| and |w1|² + |w2|² + α² = |w1|² + α²|w2|², where w1 and w2 are complex numbers and α is a real number, then
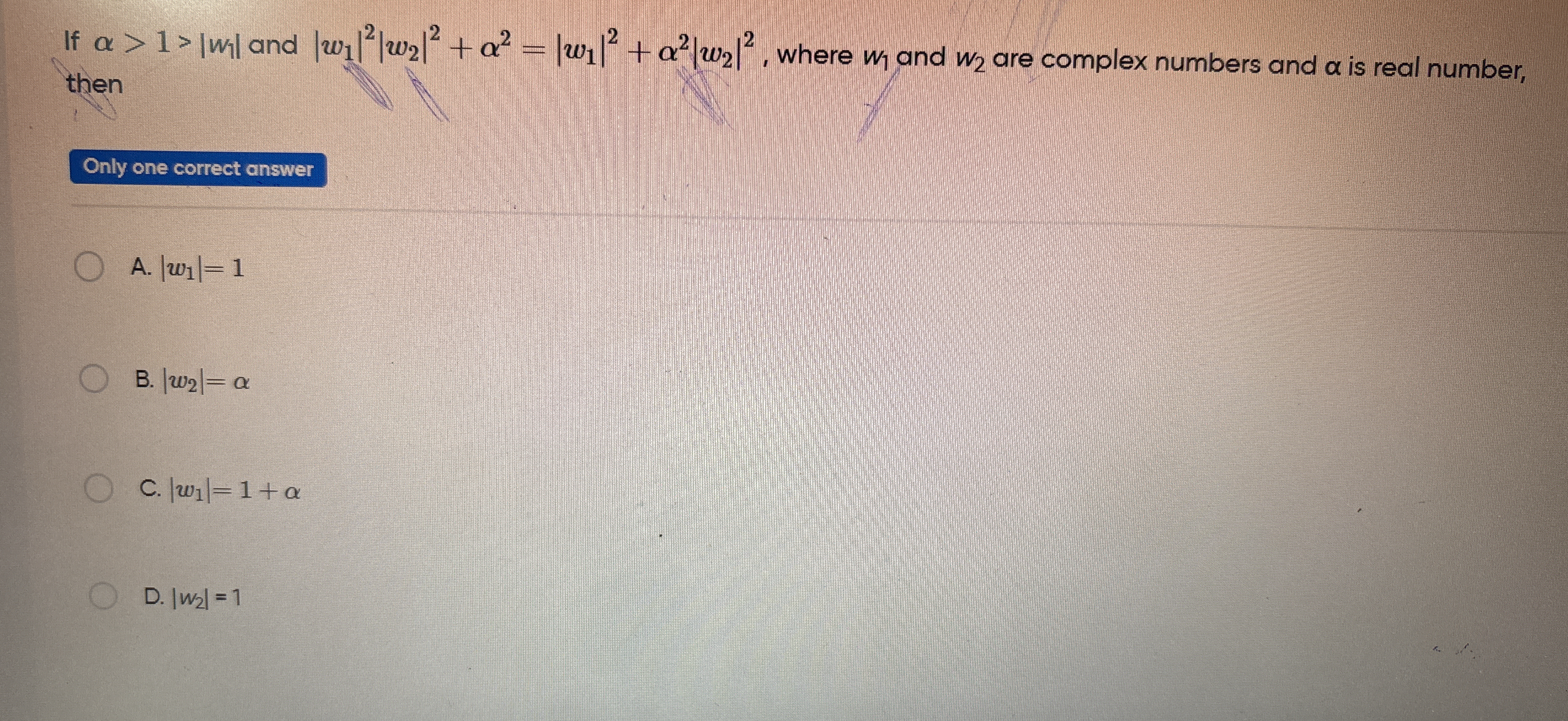
Understand the Problem
The question is asking us to evaluate the relationship between the complex numbers and their moduli under the conditions given. We need to find out which of the four provided statements about the moduli of the complex numbers is true based on the equation presented.
Answer
$|w_2| = \alpha$.
Answer for screen readers
The correct answer is: B. $|w_2| = \alpha$.
Steps to Solve
- Understand the Given Equation
We have the equation: $$ |w_1|^2 |w_2|^2 + \alpha^2 = |w_1|^2 + \alpha^2 |w_2|^2 $$
- Rearrange the Equation
Let's rearrange this equation to isolate terms related to the moduli. We subtract $|w_1|^2$ from both sides: $$ |w_1|^2 |w_2|^2 - |w_1|^2 + \alpha^2 - \alpha^2 |w_2|^2 = 0 $$
- Factor the Expression
Now, we can factor the first two terms: $$ |w_1|^2 (|w_2|^2 - 1) + \alpha^2 (1 - |w_2|^2) = 0 $$
- Set the Expression to Zero
For the equation to hold true, the two terms must sum to zero. This leads us to two possible cases:
- Case 1: $|w_2|^2 = 1$
- Case 2: $|w_1|^2 = 0$
- Analyze the Cases
If $|w_2|^2 = 1$, then $|w_2| = 1$. If $|w_1|^2 = 0$, it means $|w_1| = 0$.
Given that $\alpha > 1 > |w_1|$, we find that:
- $|w_2|$ must relate to $\alpha$ while satisfying $|w_2| \leq \alpha$.
- Check the Options
Now, we check the provided answer choices against the findings:
- Option A: $|w_1| = 1$ (incorrect because $|w_1| < 1$)
- Option B: $|w_2| = \alpha$ (could be true)
- Option C: $|w_1| = 1 + \alpha$ (incorrect, as $|w_1| < 1$)
- Option D: $|w_2| = 1$ (could be true but conflicts with given conditions on $\alpha$)
The correct answer is: B. $|w_2| = \alpha$.
More Information
The condition requires $|w_1| < 1$ and $\alpha > 1$, indicating that $|w_2|$ can be equal to $\alpha$ under these constraints. The relationship amongst $|w_1|$, $|w_2|$, and $\alpha$ reveals that while $|w_2|$ might be equal to $\alpha$, it cannot exceed it due to the conditions established.
Tips
- Assuming both $|w_1|$ and $|w_2|$ can be greater than $\alpha$.
- Misinterpreting the inequality condition on $|w_1|$. It's crucial to remember $|w_1| < 1$.
AI-generated content may contain errors. Please verify critical information