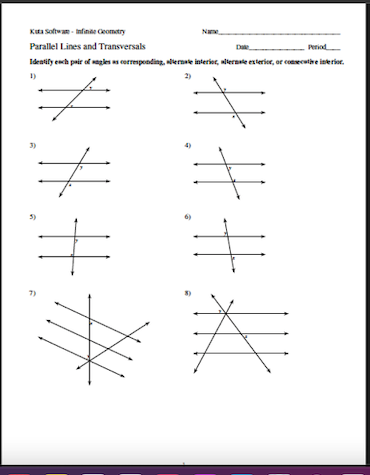
Identify each pair of angles as corresponding, alternate interior, alternate exterior, or consecutive interior.
Understand the Problem
The question is asking to identify pairs of angles formed by parallel lines and a transversal based on their relationships such as corresponding, alternate interior, alternate exterior, or consecutive interior angles.
Answer
1. Corresponding Angles 2. Alternate Interior Angles 3. Consecutive Interior Angles 4. Alternate Exterior Angles 5. Corresponding Angles 6. Consecutive Interior Angles 7. Alternate Interior Angles
Answer for screen readers
- Corresponding Angles
- Alternate Interior Angles
- Consecutive Interior Angles
- Alternate Exterior Angles
- Corresponding Angles
- Consecutive Interior Angles
- Alternate Interior Angles
Steps to Solve
-
Identify the Types of Angles
Understand the relationships between the angles formed when a transversal crosses two parallel lines. The main types are:- Corresponding Angles: Angles in the same position on parallel lines.
- Alternate Interior Angles: Angles on opposite sides of the transversal, inside the parallel lines.
- Alternate Exterior Angles: Angles on opposite sides of the transversal, outside the parallel lines.
- Consecutive Interior Angles: Angles on the same side of the transversal, inside the parallel lines.
-
Examine Each Diagram
Look at each pair of diagrams given (1 to 7) and identify the angles formed by the intersections of the transversal with the parallel lines. -
Label Each Angle Pair
For each diagram, label the angles using the terms corresponding, alternate interior, alternate exterior, or consecutive interior based on the definitions. Make sure to check their positions relative to the transversal and the parallel lines.
- Corresponding Angles
- Alternate Interior Angles
- Consecutive Interior Angles
- Alternate Exterior Angles
- Corresponding Angles
- Consecutive Interior Angles
- Alternate Interior Angles
More Information
Identifying angles formed by parallel lines and a transversal is fundamental in geometry, as it leads to understanding properties of parallel lines and transversal relationships. This knowledge is critical for solving various problems, including those involving angle measures and proofs.
Tips
- Confusing corresponding angles with alternate interior angles. Remember that corresponding angles are in the same position while alternate interior angles are across the transversal.
- Mislabeling consecutive interior angles; they are on the same side of the transversal, often leading to their identification as alternate angles.
AI-generated content may contain errors. Please verify critical information