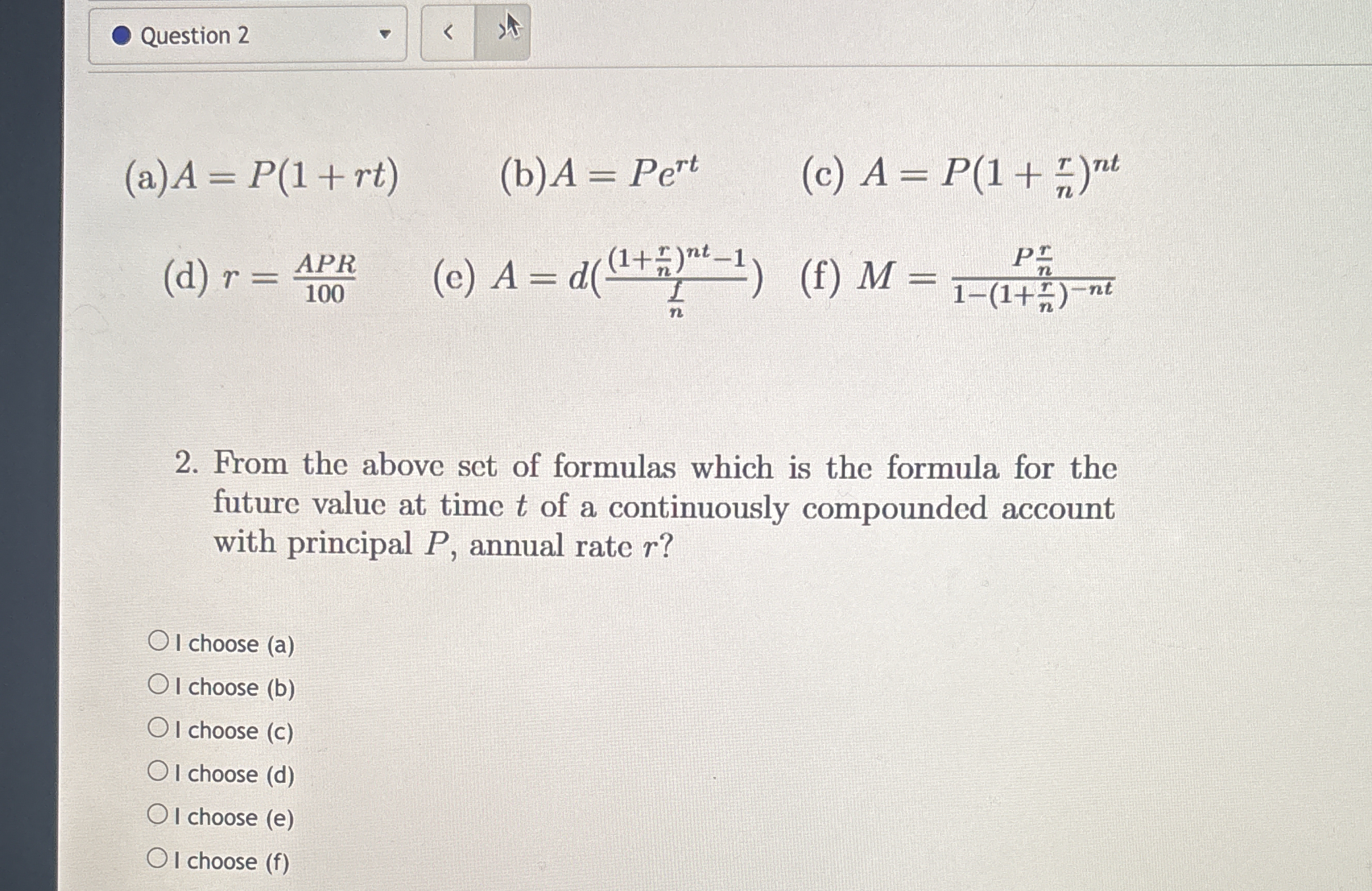
From the above set of formulas which is the formula for the future value at time t of a continuously compounded account with principal P, annual rate r?
Understand the Problem
The question is asking to identify which formula from a provided set relates to the future value of a continuously compounded account, based on specified variables such as principal and annual rate.
Answer
I choose (b) $A = Pe^{rt}$.
Answer for screen readers
The formula for the future value at time ( t ) of a continuously compounded account with principal ( P ) and annual rate ( r ) is:
$$ A = Pe^{rt} $$
Steps to Solve
- Identify the formula for continuously compounded interest
The future value ( A ) of an investment with principal ( P ), at a continuously compounded rate ( r ), after time ( t ) is given by the formula:
$$ A = Pe^{rt} $$
- Scan the provided options
We need to determine which option matches the formula for continuously compounded interest.
-
Option (a): ( A = P(1 + rt) ) (not continuous compounding)
-
Option (b): ( A = Pe^{rt} ) (this is the correct formula for continuous compounding)
-
Option (c): ( A = P(1 + \frac{r}{n})^{nt} ) (discrete compounding)
-
Option (d): ( r = \frac{APR}{100} ) (not relevant)
-
Option (e): ( A = d \left( \frac{(1 + \frac{r}{n})^{nt} - 1}{\frac{1}{n}} \right) ) (related to discrete compounding)
-
Option (f): ( M = \frac{P \cdot \frac{r}{n}}{1 - (1 + \frac{r}{n})^{-nt}} ) (not related)
- Choose the correct option
Based on the above analysis, option (b) ( A = Pe^{rt} ) is the correct choice.
The formula for the future value at time ( t ) of a continuously compounded account with principal ( P ) and annual rate ( r ) is:
$$ A = Pe^{rt} $$
More Information
The formula for continuously compounded interest shows how the principal amount grows over time when interest is calculated continuously rather than at specified intervals. This method of compounding leads to a higher final amount compared to discrete compounding methods.
Tips
- Choosing the wrong formula: Confusing continuous compounding with discrete compounding concepts can lead to selecting the wrong option. Ensure to double-check the definitions.
- Misunderstanding the variables: Not recognizing that ( r ) is the annual rate and that ( t ) must be in the same time unit as the rate can cause errors in calculations.
AI-generated content may contain errors. Please verify critical information