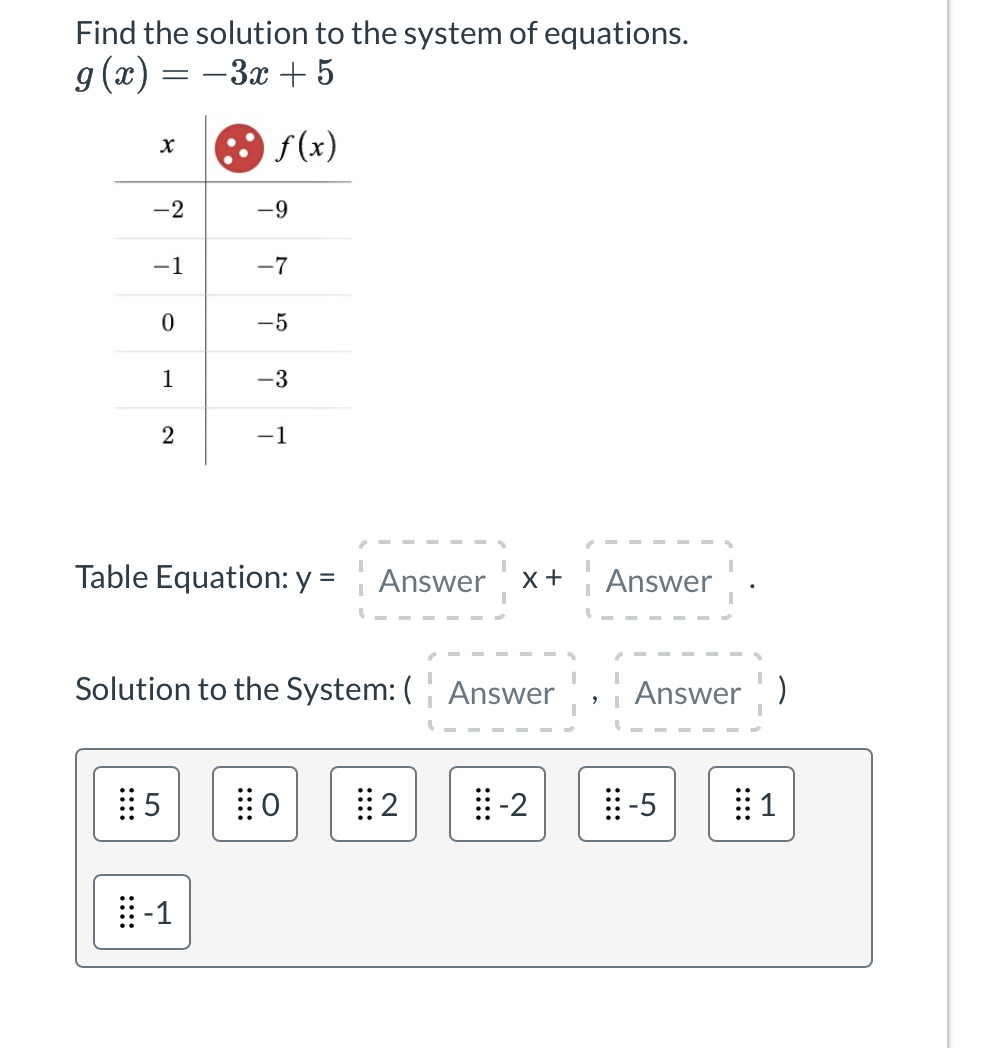
Find the solution to the system of equations g(x) = -3x + 5.

Steps to Solve

1. Identify the Points from the Table

From the given table, we extract the values of ( f(x) ) for the corresponding ( x ) values:

• At ( x = -2, f(-2) = -9 )
• At ( x = -1, f(-1) = -7 )
• At ( x = 0, f(0) = -5 )
• At ( x = 1, f(1) = -3 )
• At ( x = 2, f(2) = -1 )

2. Determine the Slope of the Line ( f(x) )

To find the slope ( m ) of the line represented by the points, we can choose two points, for example, ( (-2, -9) ) and ( (2, -1) ).

Using the slope formula:

$$ m = \frac{y_2 - y_1}{x_2 - x_1} $$

Substituting:

$$ m = \frac{-1 - (-9)}{2 - (-2)} = \frac{-1 + 9}{2 + 2} = \frac{8}{4} = 2 $$

3. Formulate the Equation of Line ( f(x) )

Now, we can use the slope-intercept formula ( y = mx + b ). We know ( m = 2 ). To find ( b ), we can use one of the points, say ( (0, -5) ):

$$ -5 = 2(0) + b $$

This gives us ( b = -5 ).

Thus, the equation of the line is:

$$ f(x) = 2x - 5 $$

4. Set the Equations Equal to Find the Intersection

Now, set ( g(x) ) equal to ( f(x) ):

$$ -3x + 5 = 2x - 5 $$

5. Solve for ( x )

Combine like terms:

$$ 5 + 5 = 2x + 3x $$

This simplifies to:

$$ 10 = 5x $$

Now solve for ( x ):

$$ x = \frac{10}{5} = 2 $$

6. Find the Corresponding ( y ) Value

Substituting ( x = 2 ) into either function to find ( y ):

Using ( g(x) ):

$$ g(2) = -3(2) + 5 = -6 + 5 = -1 $$

7. Write the Solution Point

The point where the two functions intersect is:

$$ (2, -1) $$